GoldFocus - Focus Techniques
Following is a discussion and comparison of the most commonly used focusing techniques. Discussion of each technique includes an image of the mask used, if any, and a star image with that mask in-place with near-perfect focus.
See Feature Comparison and Resolution for more information about the features of these focusing techniques and their resolving power in practice.
GoldFocus Focusing System
The GoldFocus High-Precision Mask (shown at the right), GoldFocus Plus Collimation Mask, and GoldFocus Analysis Software are designed to measure an explicit objective quality of focus. The GoldFocus Analysis Software displays the objective measure of the image's quality of focus on the astronomer's computer screen in real-time, so that there is no guesswork regarding focus.
The image at the far right is a snapshot of the GoldFocus Analysis Software which shows the measured quality of focus real-time to assist in focus adjustment.
Advantage: The quality of focus is objective, very accurate, not biased by diffraction limits nor astronomical seeing, and displayed real-time to the astronomer.
Disadvantage: Requires the GoldFocus Mask and Analysis Software.
"By Eye"
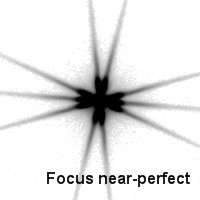
The "By Eye" technique here means an unassisted focusing method where the astronomer relies solely upon experience and judgment to achieve good focus. The "By Eye" technique uses no aperture mask and the astronomer views unobstructed images of a star or stars.
The "By Eye" technique attempts to adjust focus to make the star image(s) as small and sharp as possible. The notion of a small star image is based upon the fact that diffraction, astronomical seeing, and defocus all increase the size of a star image. Given that the diffraction limits of the telescope and camera's optical setup are fixed and assuming that astronomical seeing varies only slightly while focusing, improving focus will decrease the star image size. As a secondary criteria, the visibility of very faint stars is used as a confirmation of good focus.
Advantage: Requires no mask or special software.
Disadvantage: Technique is subjective and accuracy is greatly diminished by the effects of diffraction limits and astronomical seeing which obscures the effects of poor focus.
Hartmann (Scheiner) Mask
The Hartmann or Scheiner mask has 2-4 round holes equally spaced around the aperture of the telescope (a 3-hole version is shown at the right). Some authors distinguish a Scheiner mask as having 2 holes and a Hartmann mask as having more than 2 holes, which is perhaps historically correct.
Regardless, the number of holes does not
change the use of the mask. When severely
defocused a star image will
split into the same number of separate star images as there are
holes. When focus is good, the separate star images merge into
a single star image. Once reasonably good focus is achieved,
the task of achieving near-perfect focus is essentially the same as
the "By Eye" technique. Namely, the Hartmann (Scheiner) mask
technique ultimately relies upon experience and judgment to minimize
the size of the star image.
One of the round holes may be replaced by a triangle, as in the example shown above-right. When severely defocused a star image will split into separate star images where one is triangular shaped. The triangular shape is used as a directional aid for adjusting focus in or out. Once good focus is achieved, the triangular shape is less discernible and the use of the mask is identical to the standard round-hole Hartmann mask.
Advantage: Mask is easily homemade.
Disadvantage: Technique is subjective and accuracy is greatly diminished by the effects of diffraction limits and astronomical seeing which obscures the effects of poor focus.
Diffraction Spike Mask
A diffraction spike mask (also known as diffraction cross mask and by other names) consists of two bands or strips perpendicular to each other crossing the telescope aperture (shown at the right). Variations of this mask use slits instead of strips. The diffraction spike mask produces a star image similar to that of Newtonian diffraction spikes and for the same reasons (refer to Diffraction Theory).
When severely defocused, the diffraction fringes split into pairs of parallel fringes. With good focus, the pairs of parallel fringes merge together and appear to be a single fringe. Achieving near-perfect focus requires experience and judgment to determine when the pairs of parallel fringes are exactly coincident with each other.
Advantage: Mask is easily homemade.
Disadvantage: Technique is subjective and accuracy is diminished by the effects of astronomical seeing which blurs the distinction of when the parallel fringes merge into coincident fringes.
Modified Hartmann Mask
A Hartmann mask (see above) of 3-4 holes can be modified by replacing some or all of the round holes with triangular or square holes. A modified Hartmann mask with 3 triangles is shown at the right.
If only some of the round holes are replaced (usually by a triangle in this case), then the mask behaves much like a standard Hartmann mask. See the discussion of the standard Hartmann Mask, above.
If all round holes are replaced by triangles or squares, then the image produces various numbers of diffraction fringes depending upon the number and shape of the holes. Focusing is then similar to the Diffraction Spike Mask technique, above.
Advantage: Mask is easily homemade.
Disadvantage: Technique is subjective and accuracy is diminished by the effects of astronomical seeing which blurs the distinction of when the parallel fringes merge into coincident fringes.
Bahtinov Mask
A Bahtinov mask, so called after a Russian amateur astronomer credited with its development, consists of three sets of parallel and evenly spaced slits (shown at the right). The number, width, and angles of the slits may vary, as may the size of the three sectors of these slits.
The Bahtinov mask creates a distinctive diffraction pattern of two crossing diffraction fringes with a third diffraction fringe in-between. When defocused, the third diffraction fringe will move away from the center of the two crossing fringes. When near-perfectly focused, the third diffraction fringe will be centered between the two crossing fringes. Achieving near-perfect focus requires experience and judgment to determine when the third diffraction fringe is well-centered.
Advantage: Mask is easily homemade and commercially available.
Disadvantage: Technique is subjective and accuracy is limited by astronomer's ability to visually and accurately distinguish fringe locations.
Minimum FWHM
Minimum HFD
Minimum Sigma
All three of FWHM, HFD, and Sigma are quantitative measures of the size of a star image.
The FWHM, meaning full-width at half-maximum, is the diameter of the circle around the center of the star image where the photon flux has fallen to half of the brightest intensity of the star image.
The HFD, meaning half flux diameter, is the diameter of the circle around the center of the star image which contains half of the total photon flux of the entire star image.
The Sigma (also known as star sigma or Gaussian sigma) is the standard deviation of the star image when statistically fitted to a two-dimensional Gaussian distribution.
Each of the Minimum FWHM, HFD, and Sigma techniques are similar to the "By Eye" technique in not using a mask and in trying to minimize the size of the star image. In addition, the Minimum FWHM, HFD, and Sigma techniques use a computer analysis of the star image to measure the star image size as the FWHM, HFD, and Sigma quantity, respectively. These respective star image sizes are displayed to the astronomer by computer software, usually real-time, to assist in adjusting focus to achieve the smallest star image size.
The example screen shot above-right shows how FWHM might be displayed real-time by computer software.
Advantage: The focus measures are quantitative and objective.
Disadvantage: The quantitative measures include the effects of diffraction limits and astronomical seeing. The effects of defocus when approaching near-perfect focus are only a very small fraction of the overall measures of FWHM, HFD, and Sigma which makes these focus measures inaccurate against the background of the much larger effects of diffraction and seeing. For further information, see Resolving Power.
