Critical Focus Zone - Traditional
The traditional Critical Focus Zone must be commended for laying the foundations for the analysis of the sensitivity of the effects of focus to small movements of a focuser draw tube.
What follows calls into question the original results of the Critical Focus Zone, but does not question the basic principle that focus is amenable to theoretical analysis for the practical benefit of astronomers, astroimagers, and astrophotographers. The following should be viewed as moving the theory of the traditional Critical Focus Zone to the next, more advanced stage of understanding, as has been done with the New Critical Focus Zone. It is a natural progression to build upon the foundations given to us by the traditional Critical Focus Zone, for which we should be grateful.
The basic foundation of the traditional Critical Focus Zone is that when at perfect focus, very small changes in focus do not degrade image quality in any significant way.
The traditional form of the Critical Focus Zone states that when the defocus of a point source of light is smaller than the first dark diffraction ring of the Airy disk, then that defocus will not be measureable in an image.
The traditional Critical Focus Zone is the range of travel on the focuser draw tube within which the defocus of a point source of light is smaller than the first dark diffraction ring of the Airy disk. The traditional Critical Focus Zone (denoted CFZ) is given by the formula:
CFZ = 4.88 · λ · f 2
CFZ
- Traditional Critical Focus Zone (micrometers)
λ
- wavelength of light (micrometers)
f
- effective imaging system f/ratio (unitless)
4.88
- constant (unitless)
Important Note:
For those readers interested in using the CFZ formula, CFZ has been
superseded by the
New Critical Focus Zone.
The derivation of the formula is fairly simple when it is considered in the following way:
CFZ = 2 · (2.44 · λ · f) · f
The diameter of the first dark diffraction ring of the Airy disk is 2.44·λ·f, which is the middle term above. The f/ratio f, the last term above, scales the physical linear size of the first dark diffraction ring on the CCD imaging surface to the travel distance of the focuser draw tube. The factor of 2, the first term above, doubles the travel distance because the defocus can be either in or out by that amount.
Fallacies of the Traditional Critical Focus Zone
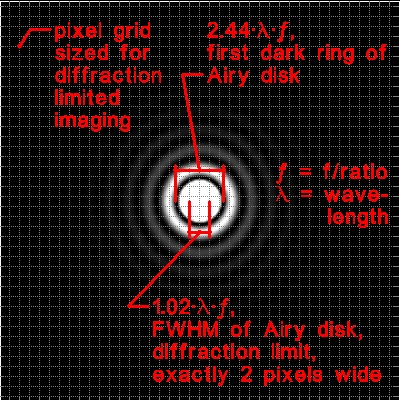

The first fallacy of the traditional Critical Focus Zone is the premise that a defocused image the size of the first dark diffraction ring of the Airy disk cannot be measured.
By the definition of the telescope diffraction limit and Critical Sampling (see the image at the right), both of which are very well established and widely accepted, any image detail greater than the FWHM of the Airy disk is measurable.
The FWHM of the Airy disk is 1.02·λ·f whereas the diameter of the first dark diffraction ring is 2.44·λ·f. The diameter of the first dark diffraction ring of the Airy disk is over twice as large (by a factor of 2.44 / 1.02) as the FWHM of the Airy disk and therefore anything defocused to that size must be measurable. The premise that it is not measurable is a direct contradiction to the definitions of the diffraction limit of a telescope and Critical Sampling.
In all likelihood, the use of the 2.44 factor in the traditional Critical Focus Zone formula was an accidental error in the beginning which has now been perpetuated by unquestioning repetition.
The second fallacy of the traditional Critical Focus Zone is that a point source of light is being defocused.
In any imaging system with a circular aperture, any defocus applies to the Airy disk and not to a point source of light.
The defocus of an Airy disk always produces an image larger and more spread than the Airy disk itself.
Defocus by the amount described by the traditional Critical Focus Zone will produce an image of the Airy disk with a FWHM over 2.2 times greater that the original Airy disk, as shown in the image to the right.
The defocused Airy disk image is therefore greater than the minimum required for measurement by the diffraction limit of the telescope and Critical Sampling by a factor of over 2.2 times and, in reality, is very severely out of focus.
Either of these two fallacies is sufficient to invalidate the formula for the traditional CFZ.
Remedies for the Traditional Critical Focus Zone
The first fallacy of the traditional Critical Focus Zone is a clerical error which could be easily remedied. However, the second fallacy of the traditional Critical Focus Zone is considerably more complex to remedy.
The difference between defocusing a point source of light, as assumed by the traditional Critical Focus Zone, and defocusing an Airy disk, as required by the actual circumstances, is a very significant difference. Fortunately, the analysis of a defocused Airy disk is one special case of the Extended Nijboer-Zernike theory - literally what the theory was designed to do.
Furthermore, although the analysis of diffraction limited cases is always the starting point of any analysis of imaging systems, the inclusion of astronomical seeing in the analysis would have practical benefit to astronomers and astroimagers. The traditional Critical Focus Zone does not address astronomical seeing in any way. The inclusion of astronomical seeing with the required defocus of an Airy disk goes a step beyond even the Extended Nijboer-Zernike theory in its complexity.
GoldAstro has undertaken research into a replacement for the traditional Critical Focus Zone. The results of that research are the New Critical Focus Zone.